Inclusion-exclusion principle proof
WebSection 3.3 Principle of Inclusion & Exclusion; Pigeonhole Principle 4 Example: Inclusion and Exclusion Principle Example 1: How many integers from 1 to 1000 are either multiples of … The inclusion exclusion principle forms the basis of algorithms for a number of NP-hard graph partitioning problems, such as graph coloring. A well known application of the principle is the construction of the chromatic polynomial of a graph. Bipartite graph perfect matchings See more In combinatorics, a branch of mathematics, the inclusion–exclusion principle is a counting technique which generalizes the familiar method of obtaining the number of elements in the union of two finite sets; symbolically … See more Counting integers As a simple example of the use of the principle of inclusion–exclusion, consider the question: How many integers … See more Given a family (repeats allowed) of subsets A1, A2, ..., An of a universal set S, the principle of inclusion–exclusion calculates the number of elements of S in none of these subsets. A generalization of this concept would calculate the number of elements of S which … See more The inclusion–exclusion principle is widely used and only a few of its applications can be mentioned here. Counting derangements A well-known application of the inclusion–exclusion principle is to the combinatorial … See more In its general formula, the principle of inclusion–exclusion states that for finite sets A1, …, An, one has the identity This can be compactly written as or See more The situation that appears in the derangement example above occurs often enough to merit special attention. Namely, when the size of the intersection sets appearing in the formulas for the principle of inclusion–exclusion depend only on the number of sets in … See more In probability, for events A1, ..., An in a probability space $${\displaystyle (\Omega ,{\mathcal {F}},\mathbb {P} )}$$, the inclusion–exclusion principle becomes for n = 2 See more
Inclusion-exclusion principle proof
Did you know?
Webemphasizes computation, problem solving, and proof technique. In particular, the book places special emphasis the Principle of Inclusion and Exclusion and the Multiplication Principle. To this end, exercise sets are included at the end of every section, ranging from simple computations (evaluate a formula for a WebMar 11, 2024 · The inclusion-exclusion principle is an important combinatorial way to compute the size of a set or the probability of complex events. It relates the sizes of individual sets with their union. Statement The verbal formula The inclusion-exclusion principle can be expressed as follows:
WebDiscrete Mathematics and Its Applications, Fifth Edition 1 The Foundations: Logic and Proof, Sets, and Functions 1.1 Logic 1.2 Propositional Equivalences 1.3 Predicates and Quantifiers 1.4 Nested Quantifiers 1.5 Methods of Proof 1.6 Sets 1.7 Set Operations 1.8 Functions 2 The Fundamentals: Algorithms, the Integers, and Matrices 2.1 Algorithms 2.2 The Growth of … WebProof Consider as one set and as the second set and apply the Inclusion-Exclusion Principle for two sets. We have: Next, use the Inclusion-Exclusion Principle for two sets on the first term, and distribute the intersection across the union in the third term to obtain: Now, use the Inclusion Exclusion Principle for two sets on the fourth term to get: Finally, the set in …
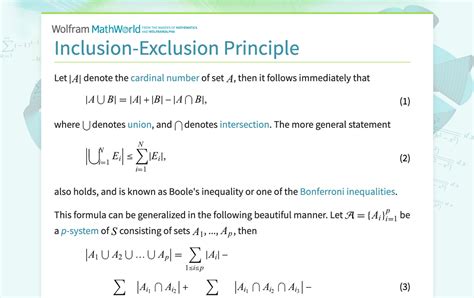
WebThis paper proposes a new closed-loop observer-based active fault diagnosis (AFD) framework using a bank of set-valued observers (SVOs). Each SVO is d… WebMar 24, 2024 · Inclusion-Exclusion Principle Contribute To this Entry » Let denote the cardinal number of set , then it follows immediately that (1) where denotes union, and denotes intersection . The more general statement (2) also holds, and is known as Boole's inequality or one of the Bonferroni inequalities .
WebAug 1, 2024 · Exclusion Inclusion Principle Induction Proof combinatorics induction inclusion-exclusion 16,359 A big hint is to prove the result for three sets, A 1, A 2, A 3, given the result for two sets. I assume you have already seen the result for two sets: A 1 ∪ A 2 = A 1 + A 2 − A 1 ∩ A 2 So what do we get with three sets?
Webthat the inclusion-exclusion principle has various formulations including those for counting in combinatorics. We start with the version for two events: Proposition 1 (inclusion-exclusion principle for two events) For any events E,F ∈ F P{E∪F} = P{E}+P{F}−P{E∩F}. Proof. dunk aestheticWeb1 Principle of inclusion and exclusion. Very often, we need to calculate the number of elements in the union of certain sets. Assuming that we know the sizes of these sets, and … dunk and associates canadaWebOnline courses with practice exercises, text lectures, solutions, and exam practice: http://TrevTutor.comWe introduce the inclusion-exclusion principle.Visit... dunk air forceWebThe Principle of Inclusion-Exclusion (abbreviated PIE) provides an organized method/formula to find the number of elements in the union of a given group of sets, the … dunk acg terraWebProve the following inclusion-exclusion formula. P ( ⋃ i = 1 n A i) = ∑ k = 1 n ∑ J ⊂ { 1,..., n }; J = k ( − 1) k + 1 P ( ⋂ i ∈ J A i) I am trying to prove this formula by induction; for n = 2, let … dunk and bright credit cardWeb1 Principle of inclusion and exclusion Very often, we need to calculate the number of elements in the union of certain sets. Assuming that we know the sizes of these sets, and their mutual intersections, the principle of inclusion and exclusion allows us to do exactly that. Suppose that you have two setsA;B. dunk and bright financingWebThe Inclusion-Exclusion Principle From the First Principle of Counting we have arrived at the commutativity of addition, which was expressed in convenient mathematical notations as … dunk and bright full mattress